Tony Bomford's Marvelous Mathematical Rugs

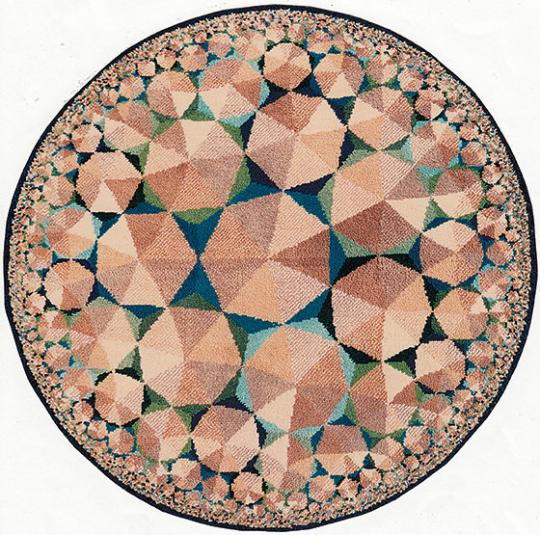
Heptagons and Triangles, 7' diameter, 62,575 knots. Designed and latch hooked by Tony Bomford, 1988. This is the second of two rugs based on a conformal projection of a quasi-regular tessellation of heptagons and triangles from the hyperbolic plane on to the Euclidean plane.


Anthony Gerald Bomford (Tony; 1927–2003) was an engineer, surveyor, mathematician, mapmaker, and—in his spare time—a rug hooker. While at first rug hooking may seem somewhat out of place in this list of esteemed accomplishments, it is in fact inextricably linked to his extraordinary life. 

Tony created his unique collection of 18 rugs using the latch-hooking method, with a latch hook tool and short, precut pieces of woolen yarn to create a long knotted pile on an even-weave rug canvas base. Latch hooking was incredibly popular in Australia during the 1970s and 1980s. (Even today, when I tell people I hook rugs, they almost always assume I am referring to latch hook rugs. Most Australians are unfamiliar with traditional loop pile rugs.) 

With his sharp mathematical brain (Tony studied specialist mathematics at Cambridge University), it is understandable why this methodical style of rug hooking would have appealed to him. In fact, Needlework Through History: An Encyclopedia cites a teacher in Brooklyn, New York, who used the latch hooking geometric grid to teach mathematical concepts to students.

This article is from the January/February 2015 issue. For more information on our issues, check out our issues page.